Shown below is the second shortest published math paper; it’s the shortest published math paper, except for this one. This paper relates to an extension to Fermat’s last theorem. That’s well known math, though I think a few words of background would help the educated lay reader.

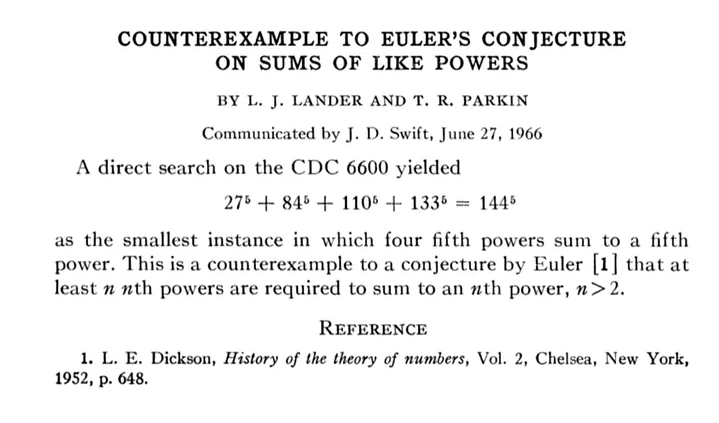
By way of background, Fermat’s last theorem states that there is no set of integers for which Aⁿ + Bⁿ = Qⁿ, where n is an integer larger than 2. Thus, there is no set where A³ + B³ = Q³ or A⁵ + B⁵ = Q⁵, etc. This theorem was really a conjecture until recently though Fermat claimed to have proven it in 1695.

The Euler conjecture of the title here, is related to Fermat’s conjecture/theorum: Either conjectured that the smallest collections of A, B, C, D.., whose power to the n, summed, will equal some whole number to the power n, Qⁿ , must have at least as many components (A,B,C,D,..) as the exponent value, n. Thus, while you might find a set of five numbers, A, B, C, D, E where A⁵ + B⁵ + C⁵ + D⁵ + E⁵ = Q⁵, you can’t find a set of four numbers where A⁵ + B⁵ + C⁵ + D⁵ = Q⁵. The paper above disproves this conjecture in a most clear way; it shows a counter-example where A⁵ + B⁵ + C⁵ + D⁵ = Q⁵.

This is, in a sense, the ideal math paper: clear, short, important, and true. For background to this conjecture, the authors merely reference a page of a math history book.

Robert E. Buxbaum, March 17, 2025. The shortest paper ever is this gem in the journal of psychology.